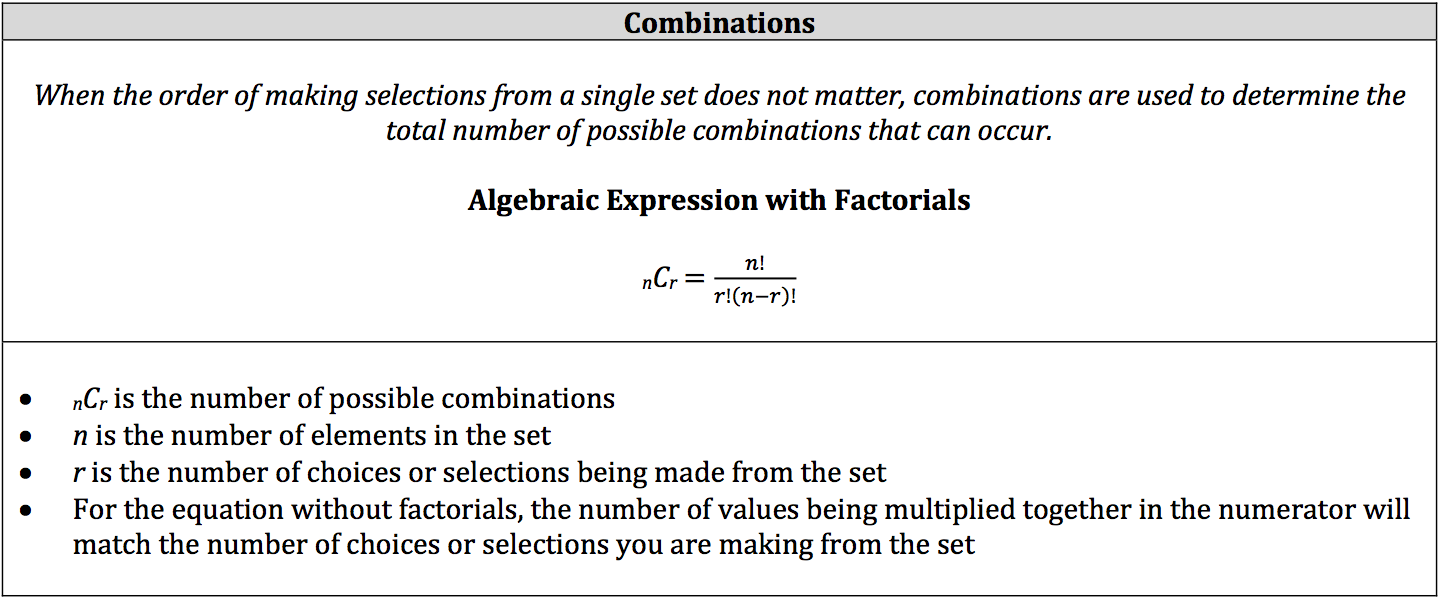
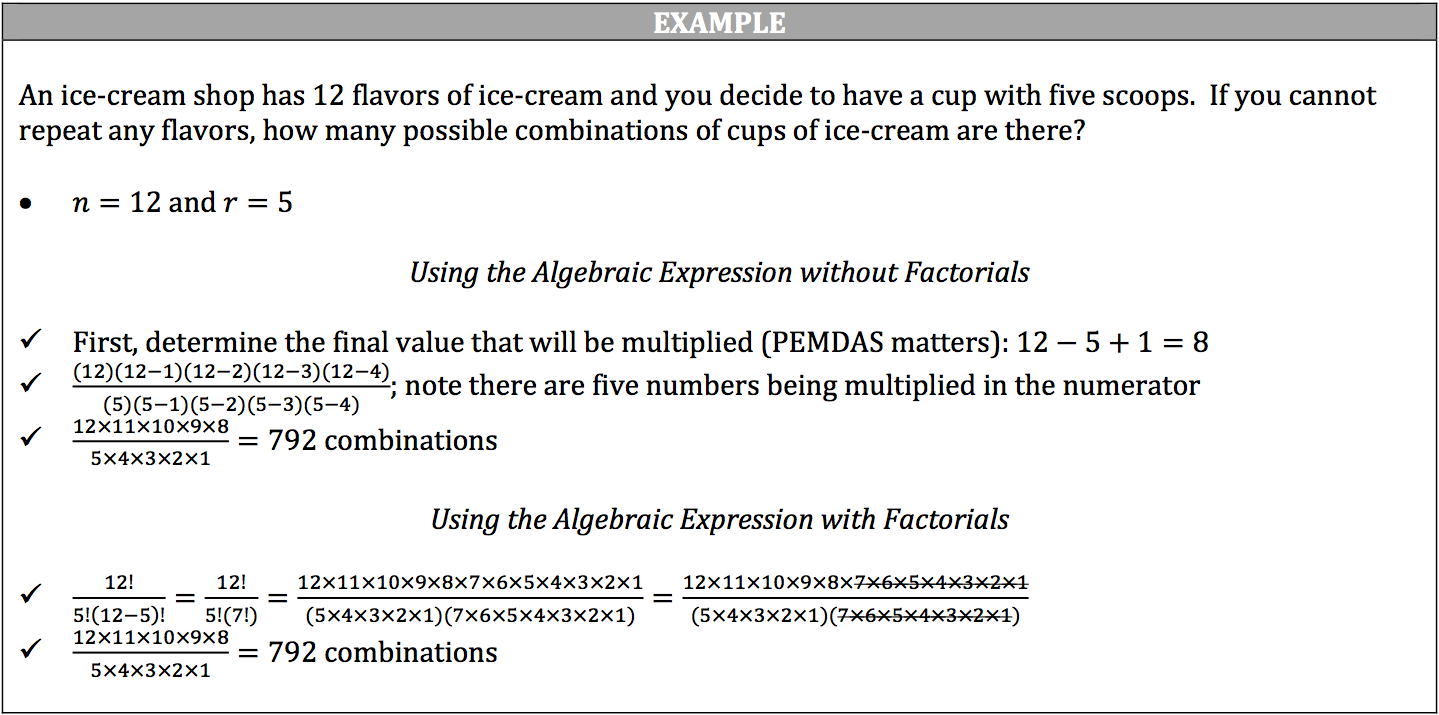
Combinations, another variation of the fundamental counting principle, come into play when the order of the selected elements from the single set does not matter. For example, the ice‐cream store has a special for a cup of ice‐cream with five flavors (no toppings), but you cannot choose the same flavor again for any of the five scoops. Choosing chocolate for the first scoop and vanilla for the second scoop is not any different than choosing vanilla first and chocolate second—you still have a scoop of chocolate and a scoop of vanilla in your cup after the first two selections.





Leave A Comment